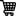The circular measure of an angle of an isoceles triangle is 5π/9. Circular measure of one of the other angles must be
The circular measure of an angle of an isoceles triangle is 5π/9. Circular measure of one of the other angles must be
[A]$latex \frac{4\pi }{9}&s=1$
[B]$latex \frac{2\pi }{9}&s=1$
[C]$latex \frac{5\pi }{9}&s=1$
[D]$latex \frac{5\pi }{18}&s=1$
$latex \frac{2 \pi}{9}&s=1$
Sum of remaining two angles = $latex \pi -\frac{5\pi }{9}=\frac{4\pi}{9}&s=1$
∴ Each angle = $latex \frac{1}{2}\times \frac{4\pi }{9}=\frac{2\pi }{9}&s=1$
Hence option [B] is the right answer.
